The concept of power kinetic energy over time is a fundamental aspect of physics and engineering, playing a crucial role in understanding the behavior of objects in motion. At its core, kinetic energy is the energy an object possesses due to its motion, while power is the rate at which energy is transferred or converted. The relationship between these two quantities is intimately linked, with power being the derivative of energy with respect to time. In this article, we will delve into the intricacies of power kinetic energy over time, exploring its principles, applications, and implications.
Key Points
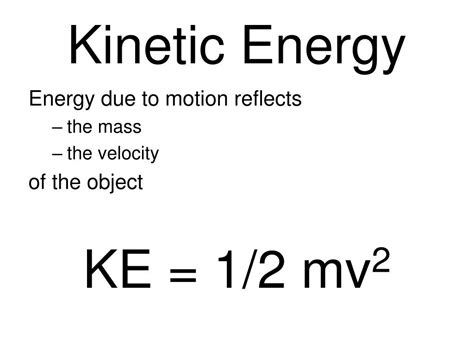
- Kinetic energy is directly proportional to the square of an object's velocity, as described by the equation KE = 0.5mv^2, where m is the mass and v is the velocity.
- Power, as the rate of energy transfer, is crucial for understanding how kinetic energy changes over time, given by the formula P = dE/dt, where E is energy and t is time.
- The work-energy theorem states that the net work done on an object is equal to its change in kinetic energy, providing a direct link between the energy transferred and the resulting motion.
- Efficiency in energy transfer is critical in maximizing the kinetic energy achieved from a given power input, with real-world applications in transportation, manufacturing, and renewable energy systems.
- Understanding the dynamics of power kinetic energy over time is essential for designing and optimizing systems, from vehicle propulsion to industrial machinery, to achieve desired performance and efficiency.
Foundational Principles of Kinetic Energy and Power
Kinetic energy, the energy of motion, is a scalar quantity that depends on the mass and velocity of an object. The kinetic energy (KE) of an object is given by the equation KE = 0.5mv^2, where m is the mass of the object and v is its velocity. This equation shows that kinetic energy is directly proportional to the square of the velocity, meaning that even small increases in velocity can result in significant increases in kinetic energy. Power, on the other hand, is a vector quantity that represents the rate at which energy is transferred or converted. It is defined as the derivative of energy with respect to time, given by the formula P = dE/dt, where E is energy and t is time.
Work-Energy Theorem and Its Implications
The work-energy theorem provides a fundamental link between the net work done on an object and its change in kinetic energy. The theorem states that the net work done on an object is equal to its change in kinetic energy (W = ΔKE). This means that the energy transferred to an object through work done on it results in a change in its kinetic energy, assuming no other forms of energy are involved. This principle is crucial for understanding how power affects kinetic energy over time, as it highlights the direct relationship between the energy input (work) and the resulting kinetic energy of the object.
| Quantity | Formula | Description |
|---|---|---|
| Kinetic Energy (KE) | 0.5mv^2 | Energy an object has due to its motion |
| Power (P) | dE/dt | Rate of energy transfer or conversion |
| Work-Energy Theorem | W = ΔKE | Net work done equals change in kinetic energy |
Applications and Implications of Power Kinetic Energy
The concept of power kinetic energy over time has far-reaching implications across various fields, including transportation, manufacturing, and renewable energy. In transportation, for instance, the power output of an engine directly affects the kinetic energy of a vehicle, influencing its acceleration and overall performance. Similarly, in manufacturing, understanding how power affects kinetic energy is crucial for optimizing production processes, ensuring that machinery operates at peak efficiency to minimize energy waste and maximize output.
Efficiency and Energy Transfer
Efficiency in energy transfer is a critical factor in maximizing the kinetic energy achieved from a given power input. In real-world applications, energy losses due to friction, heat, and other factors can significantly reduce the effectiveness of energy transfer. Therefore, designing systems with high efficiency is essential to ensure that the power input translates into useful kinetic energy, rather than being dissipated as waste energy. This principle is particularly important in the development of renewable energy systems, where maximizing energy output from available power inputs is crucial for achieving sustainability and reducing environmental impact.
In conclusion, the relationship between power and kinetic energy over time is a complex and multifaceted concept that underlies many fundamental principles in physics and engineering. By understanding how power influences kinetic energy, and vice versa, we can better design and optimize systems to achieve desired performance and efficiency. This knowledge not only enhances our comprehension of the natural world but also contributes to the development of innovative technologies and sustainable solutions for the future.
What is the formula for calculating kinetic energy?
+The formula for kinetic energy is KE = 0.5mv^2, where m is the mass of the object and v is its velocity.
How does power affect kinetic energy over time?
+Power, as the rate of energy transfer, directly influences the change in kinetic energy over time. According to the work-energy theorem, the net work done on an object (which is related to power) equals its change in kinetic energy.
Why is efficiency important in energy transfer?
+Efficiency is crucial because it determines how much of the input power is converted into useful kinetic energy, rather than being lost as waste energy. High efficiency means that more of the power input is utilized effectively, leading to better performance and less energy waste.



